Note:  icosidodecahedral symmetry, a term coined (as far as I know) by George Hart, means exactly the same thing as icosahedral symmetry. I simply use the term I like better. Also, a few of these, but not many, are chiral.

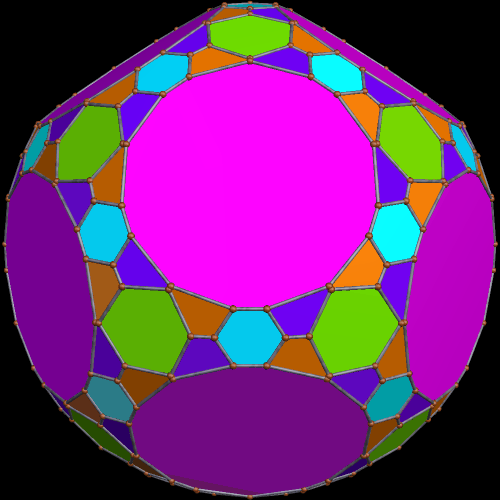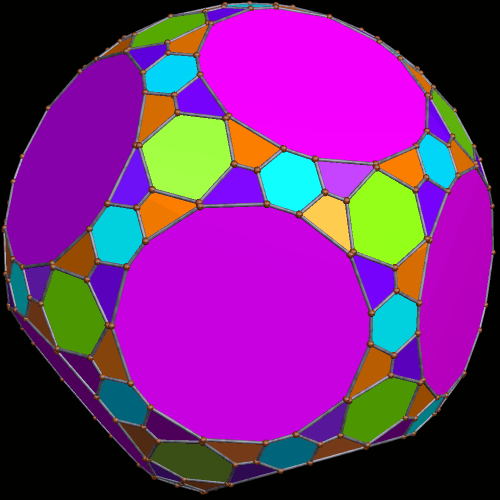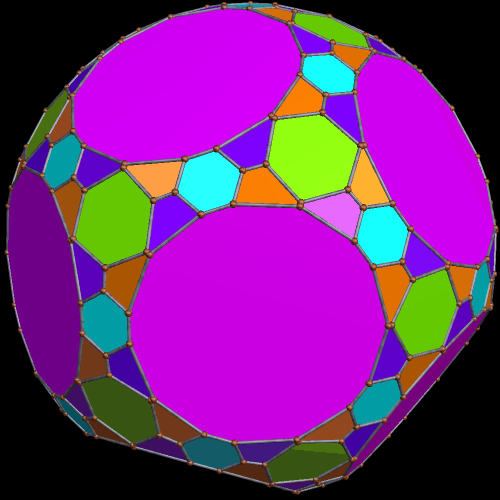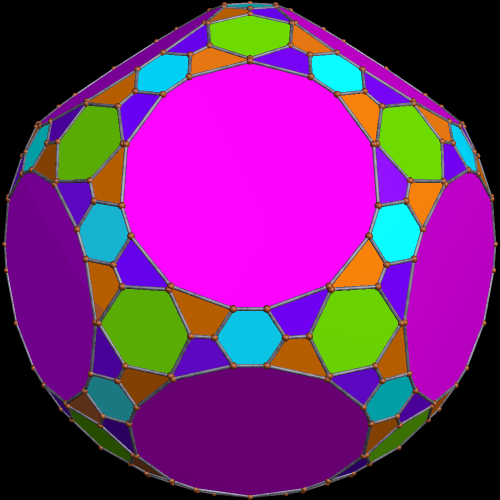
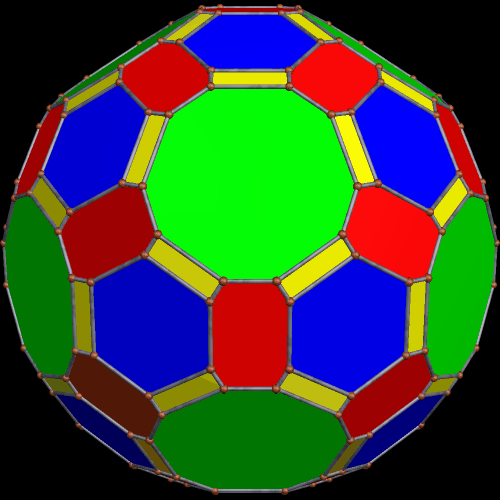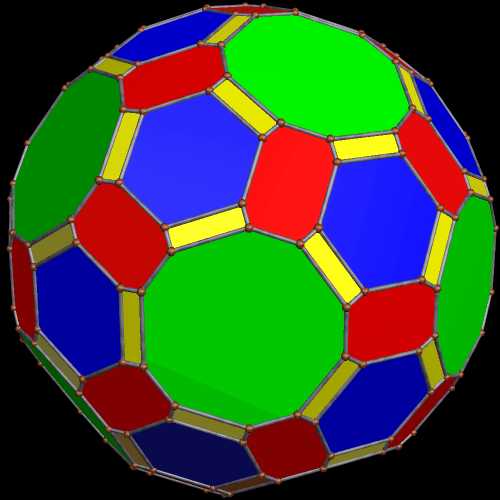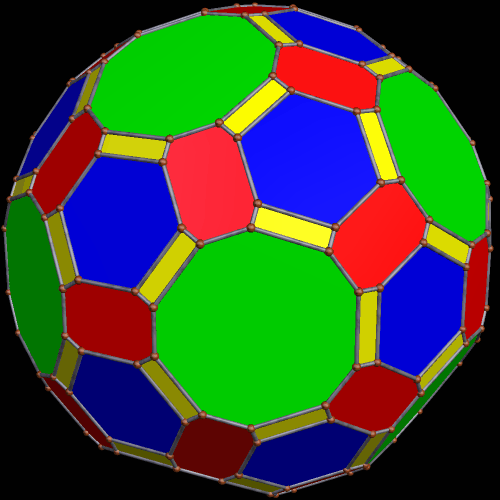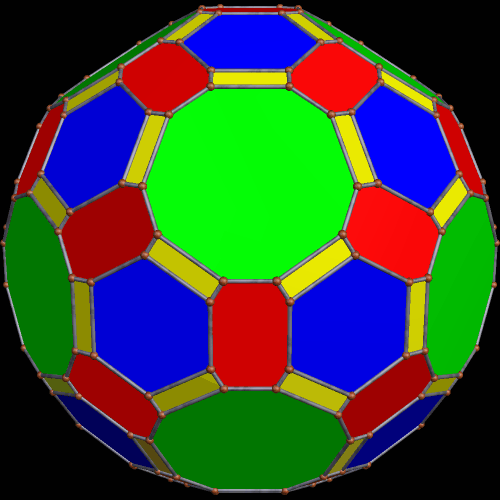
The images directly above and below show the shape of the most symmetrical 240-carbon-atom fullerene.

The image above is of the compound of five tetrahedra. This compound is chiral, and the next image is the compound of the compound above, and its mirror-image.

In the next two, I was experimenting with placing really big spheres at the vertices of polyhedra. The first one is the great dodecahedron, rendered in this unusual style, with the faces rendered invisible.

I made these using Stella 4d: Polyhedron Navigator. You may try this program for free at http://www.software3d.com/Stella.php.